Andrea Signori, PhD, AvH Fellow
Department of Mathematics
•
Politecnico di Milano
•
Via E. Bonardi 9, 20133 Milano, Italy
•
andrea.signori@polimi.it
Lake Como School of Advanced Studies (2022)
Lecture, Politecnico di Milano (2022)
Lecture, Politecnico di Milano (2023)
XXII Congresso UMI, Pisa (2023)
CIME Summer school, Cetraro (2024)
Italian-Japanese Workshop, Pavia (2024)
I am currently serving as a Non-tenure track Assistant Professor RTDa
at the Department of Mathematics of the Politecnico of Milano (Italy).
Furthermore, I am a Alexander von Humboldt Research Fellow.
In date 05-06-2023 I succeeded in the Italian National Abilitation for associate professorship.
My researches are mainly focused on phase-field approaches, optimal control theory and well-posedness analysis of nonlinear
partial differential equations and complex systems with biological applications. Among my fields of interest are: well-posedness,
regularity, asymptotic analysis, optimality control and variational approach to PDEs.
Further details can be found below.
Publications (Last Update: 04/10/24, reverse chronological order)
Recent Preprints
- P. Colli, G. Gilardi, A. Signori and J. Sprekels,
Solvability and optimal control of a multi-species Cahn–Hilliard–Keller–Segel
tumor growth model.
Preprint arXiv:2407.18162 [math.AP], (2024), 1-39. WIAS Preprint. - M. Grasselli, L. Scarpa and A. Signori,
Cahn–Hilliard equations with singular potential, reaction term and pure phase initial datum.
Preprint arXiv:2404.12113 [math.AP], (2024), 1-32.
Published Papers
- P. Colli, P. Knopf, G. Schimperna and A. Signori,
Two-phase flows through porous media described by a
Cahn–Hilliard–Brinkman model with dynamic boundary conditions.
J. Evol. Equ., 24(85) (2024), Online first.
doi.org/10.1007/s00028-024-00999-y. Preprint arXiv:2312.15274 [math.AP] - H. Garcke, K. F. Lam, R. Nürnberg and A. Signori,
Complex pattern formation governed by a Cahn–Hilliard–Swift–Hohenberg system:
Analysis and numerical simulations.
Math. Models Methods Appl. Sci., 34(11) (2024), 2055-2097.
doi.org/10.1142/S021820252450043X. Preprint arXiv:2405.01947 [math.AP] - P. Colli, G. Gilardi, A. Signori and J. Sprekels,
Curvature effects in pattern formation: well-posedness and optimal control of a sixth-order
Cahn–Hilliard equation.
SIAM J. Math. Anal., 56 (2024), 4253-5078.
doi.org/10.1137/24M1630372. Preprint arXiv:2401.05189 [math.AP]. WIAS Preprint. - A. Agosti and A. Signori,
Analysis of a multi-species Cahn–Hilliard–Keller–Segel tumor
growth model with chemotaxis and angiogenesis.
J. Differential Equations, 403 (2024), 308-367.
doi.org/10.1016/j.jde.2024.05.025. Preprint arXiv:2311.13470 [math.AP] - A. Poiatti and A. Signori,
Regularity results and optimal velocity control of the convective nonlocal
Cahn–Hilliard equation in 3D.
ESAIM Control Optim. Calc. Var., 30 (Online first) (2024).
doi.org/10.1051/cocv/2024007. Preprint arXiv:2304.12074 [math.OC] - P. Colli, G. Gilardi, A. Signori and J. Sprekels,
On a Cahn–Hilliard system with source term and thermal memory.
Nonlinear Analysis, 240 (2023), 113461.
doi.org/10.1016/j.na.2023.113461. Preprint arXiv:2207.08491 [math.AP]. WIAS Preprint. - P. Colli, G. Gilardi, A. Signori and J. Sprekels,
Optimal temperature distribution for a nonisothermal Cahn–Hilliard system in two dimensions with source term and double obstacle potential.
Ann. Acad. Rom. Sci., Ser. Math. Appl., 15 (2023), 175-204.
doi.org/10.56082/annalsarscimath.2023.1-2.175. Preprint arXiv:2303.13266 [math.OC]. WIAS Preprint. - G. Gilardi, E. Rocca and A. Signori,
Well-posedness and optimal control for a viscous Cahn–Hilliard–Oono system with dynamic boundary conditions.
Discrete Contin. Dyn. Syst. Ser. S, 16 (2023), 3573-3605.
doi/10.3934/dcdss.2023127. Preprint arXiv:2309.09053 [math.AP]. - G. Gilardi, A. Signori and J. Sprekels,
Nutrient control for a viscous Cahn–Hilliard–Keller–Segel model with logistic source describing tumor growth.
Discrete Contin. Dyn. Syst. Ser. S, 16 (2023), 3552-3572.
doi/10.3934/dcdss.2023123. Preprint arXiv:2309.09052 [math.OC] - P. Colli, G. Gilardi, A. Signori and J. Sprekels,
Optimal temperature distribution for a nonisothermal Cahn–Hilliard system with source term.
Appl. Math. Optim., 88, Online first (2023).
doi.org/10.1007/s00245-023-10039-9. Preprint arXiv:2303.00488 [math.OC]. WIAS Preprint. - P. Colli, G. Gilardi, A. Signori and J. Sprekels,
Cahn–Hilliard–Brinkman model for tumor growth with possibly singular potentials.
Nonlinearity, 36 (2023), 4470-4500.
doi.org/10.1088/1361-6544/ace2a7. Preprint arXiv:2204.13526 [math.AP]. WIAS Preprint. - H. Garcke, K. F. Lam, R. Nürnberg and A. Signori,
Phase field topology optimisation for 4D printing.
ESAIM Control Optim. Calc. Var., Online first (2023).
doi.org/10.1051/cocv/2023012. Preprint arXiv:2207.03706 [math.OC]. - H. Garcke, K. F. Lam, R. Nürnberg and A. Signori,
Overhang penalization in additive manufacturing via phase field structural topology optimization with anisotropic energies.
Appl. Math. Optim., 87(44) (2023).
doi.org/10.1007/s00245-022-09939-z. Preprint arXiv:2111.14070 [math.OC]. - M. Grasselli, L. Scarpa and A. Signori,
On a phase field model for RNA-Protein dynamics.
SIAM J. Math. Anal., 55(1) (2023), 405-457.
doi.org/10.1137/22M1483086. Preprint arXiv:2203.03258 [math.AP]. - P. Colli, G. Gilardi, A. Signori and J. Sprekels,
Optimal control of a nonconserved phase field model of Caginalp type with thermal memory and double obstacle potential.
Discrete Contin. Dyn. Syst. Ser. S, 16(9) (2023), 2305-2325.
doi.org/10.3934/dcdss.2022210. Preprint arXiv:2207.00375 [math.OC]. WIAS Preprint. - E. Rocca, G. Schimperna and A. Signori,
On a Cahn–Hilliard–Keller–Segel model with generalized logistic source describing tumor growth.
J. Differential Equations, 343 (2023), 530-578.
doi.org/10.1016/j.jde.2022.10.026. Preprint arXiv:2202.11007 [math.AP]. - P. Colli, A. Signori and J. Sprekels,
Analysis and optimal control theory for a phase field model of Caginalp type with thermal memory.
Commun. Optim. Theory, 4 (2022).
doi.org/10.23952/cot.2022.4. Preprint arXiv:2107.09565 [math.OC]. WIAS Preprint. - P. Colli, A. Signori and J. Sprekels, Optimal control problems with sparsity for phase field tumor growth models involving variational inequalities.
J. Optim. Theory Appl., 194 (2022), 25-58.
doi.org/10.1007/s10957-022-02000-7. Preprint arXiv:2104.09814 [math.OC]. WIAS Preprint. - E. Rocca, L. Scarpa and A. Signori, Parameter identification for nonlocal phase field models for tumor growth via optimal control and asymptotic analysis.
Math. Models Methods Appl. Sci., 31(13) (2021), 2643-2694.
doi.org/10.1142/S0218202521500585. Preprint arXiv:2009.11159 [math.AP]. - P. Knopf and A. Signori, Existence of weak solutions to multiphase Cahn–Hilliard–Darcy and Cahn–Hilliard–Brinkman models for stratified tumor growth with chemotaxis and general source terms.
Comm. Partial Differential Equations, 47(2) (2022), 233-278.
doi.org/10.1080/03605302.2021.1966803. Preprint arXiv:2105.09068 [math.AP]. - P. Colli, A. Signori and J. Sprekels, Second-order analysis of an optimal control problem in a phase field tumor growth model with singular potentials and chemotaxis.
ESAIM Control Optim. Calc. Var., 27 (2021).
doi.org/10.1051/cocv/2021072. Preprint arXiv:2009.07574 [math.AP]. WIAS Preprint. - L. Scarpa and A. Signori, On a class of non-local phase-field models for tumor growth with possibly singular potentials, chemotaxis, and active transport.
Nonlinearity, 34 (2021), 3199-3250.
doi.org/10.1088/1361-6544/abe75d. Preprint arXiv:2002.12702 [math.AP]. - H. Garcke, K. F. Lam and A. Signori, Sparse optimal control of a phase field tumour model with mechanical effects.
SIAM J. Control Optim., 59(2) (2021), 1555-1580.
doi.org/10.1137/20M1372093. Preprint arXiv:2010.03767 [math.OC]. - S. Frigeri, K. F. Lam and A. Signori, Strong well-posedness and inverse identification problem of a non-local phase field tumor model with degenerate mobilities.
European J. Appl. Math., 33(2) (2022), 267-308.
doi:10.1017/S0956792521000012. Preprint arXiv:2004.04537 [math.AP]. - P. Knopf and A. Signori, On the nonlocal Cahn–Hilliard equation with nonlocal dynamic boundary condition and boundary penalization.
J. Differential Equations, 280(4) (2021), 236-291.
doi.org/10.1016/j.jde.2021.01.012. Preprint arXiv:2004.00093 [math.AP]. - H. Garcke, K. F. Lam and A. Signori, On a phase field model of Cahn–Hilliard type for tumour growth with mechanical effects.
Nonlinear Anal. Real World Appl., 57 (2021), 103192.
doi.org/10.1016/j.nonrwa.2020.103192. Preprint arXiv:1912.01945 [math.AP]. -
P. Colli, A. Signori and J. Sprekels, Optimal control of a phase field system modelling tumor growth with chemotaxis and singular potentials.
Appl. Math. Optim., 83 (2021), 2017-2049.
doi.org/10.1007/s00245-019-09618-6 (see also the Erratum). Preprint arXiv:1907.03566 [math.AP]. WIAS Preprint. -
P. Colli and A. Signori, Boundary control problem and optimality conditions for the Cahn–Hilliard equation with dynamic boundary conditions.
Internat. J. Control, 94 (2021), 1852-1869.
doi.org/10.1080/00207179.2019.1680870. Preprint arXiv:1905.00203 [math.AP]. -
A. Signori, Penalisation of long treatment time and optimal control of a tumour growth model of Cahn–Hilliard type with singular potential.
Discrete Contin. Dyn. Syst. Ser. A, 41(6) (2021), 2519-2542.
doi.org/10.3934/dcds.2020373. Preprint arXiv:1906.03460 [math.AP]. -
A. Signori, Vanishing parameter for an optimal control problem modeling tumor growth.
Asymptot. Anal., 117 (2020), 43-66.
doi.org/10.3233/ASY-191546. Preprint arXiv:1903.04930 [math.AP]. -
A. Signori, Optimal treatment for a phase field system of Cahn–Hilliard type modeling tumor growth by asymptotic scheme.
Math. Control Relat. Fields, 10 (2020), 305-331.
doi:10.3934/mcrf.2019040. Preprint arXiv:1902.01079 [math.AP]. -
A. Signori, Optimality conditions for an extended tumor growth model with double obstacle potential via deep quench approach.
Evol. Equ. Control Theory, 9(1) (2020), 193-217.
doi:10.3934/eect.2020003. Preprint arXiv:1811.08626 [math.AP]. -
A. Signori, Optimal distributed control of an extended model of tumor growth with logarithmic potential.
Appl. Math. Optim., 82 (2020), 517-549.
doi.org/10.1007/s00245-018-9538-1. Preprint arXiv:1809.06834 [math.AP]. -
PhD Thesis: A. Signori, Understanding the Evolution of Tumours, a Phase-field Approach: Analytic Results and Optimal Control, 2020.
(Doctoral advisor: Prof. Pierluigi Colli, Università di Pavia).
Forthcoming Events
Italo-Japanese Workshop on Variational Perspectives for PDEs,
9-13 September 2024,
Pavia, (Italy)
Mathematics for our Health (M4H) Workshop,
7-8 November 2024,
Politecnico di Milano, (Italy)
The 14th AIMS Conference,
16-20 December 2024,
NYU Abu Dhabi, Abu Dhabi (UAE)
EMS Topical Activity Group (TAG) on Mixtures - Mixtures: Modeling, Analysis and Computing
,
5-7 February 2025,
Prague (Czech Republic)
March-May 2025: Visiting at the University of Regensburg (Humboldt Fellowship program), Germany
10-18 May 2025: Visiting at the Weierstrass Institute (WIAS), Germany
Research interests
phase-field based Tumor growth models
Cancer remains one of the foremost causes of global mortality in our modern era. Undoubtedly, comprehending the intricate process of solid tumor growth stands as one of the principal challenges of the 21st century. Within this context, mathematics holds the potential to assume a pivotal role. Multiscale mathematical modeling offers a quantitative tool that can greatly aid in diagnostic and prognostic applications. This is why nonlinear partial differential equations (PDEs) provide a tangible bridge between the experimental techniques employed by medical professionals and the more abstract realm of mathematics. Numerical solvers can be deployed as supportive tools in clinical therapies. The essence of the phase-field models that pique my interest revolves around the amalgamation of a Cahn–Hilliard type equation featuring a source term (accounting for cell-to-cell adhesion effects) with a reaction-diffusion equation describing the behavior of surrounding species that serve as nutrients.
Biological models and chemotaxis
Recently, phase separation has emerged as a fundamental concept in the field of Cell Biology, particularly concerning
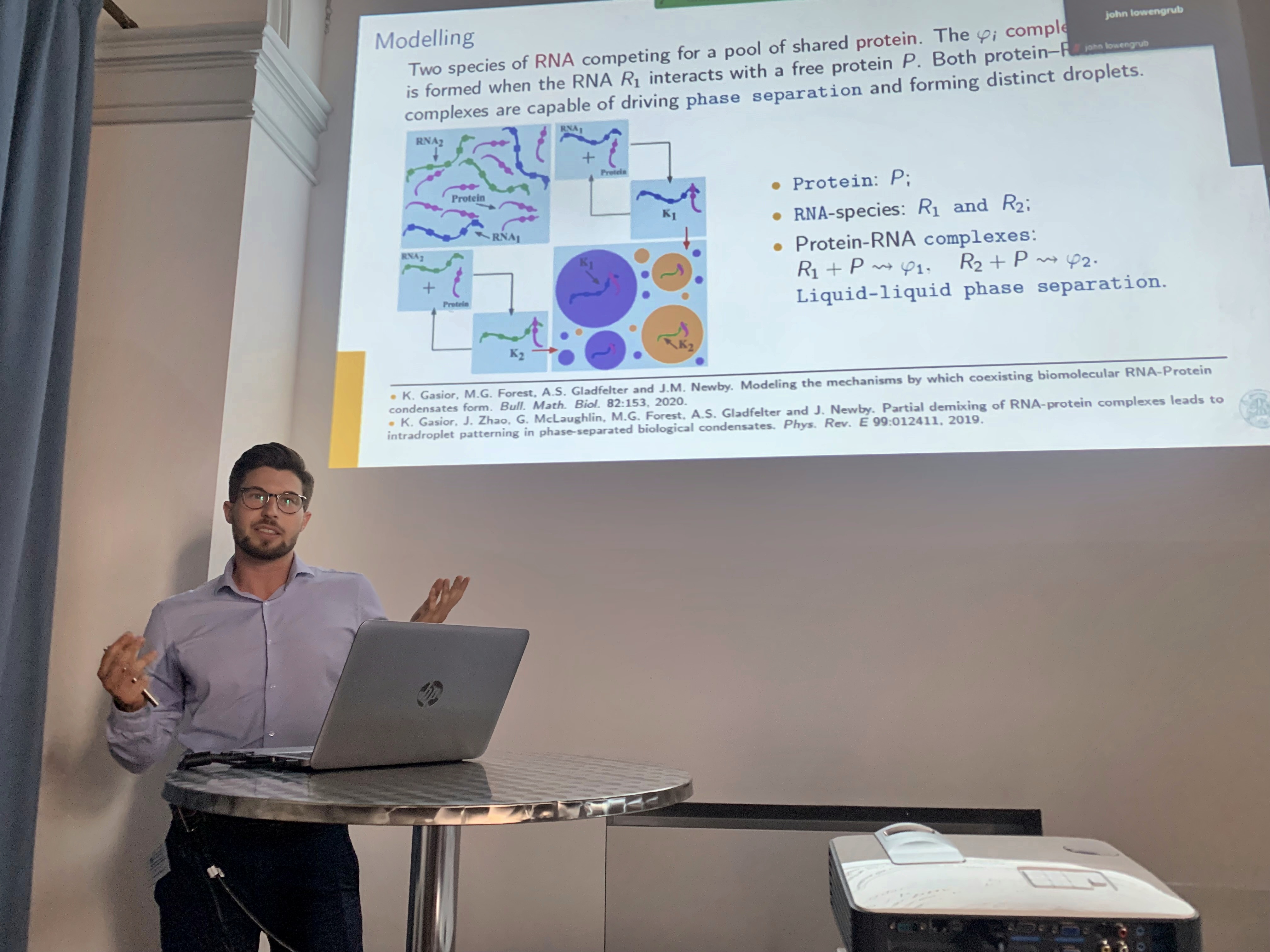
intracellular organization.
In this direction, protein-RNA complexes models play a significant and significant role. These models consist of a protein, two different RNA species, and two distinct protein-RNA complexes. The interaction between the protein and RNA species in a specific solvent is described by a interconnected set of reaction-diffusion equations, with the reaction terms being dependent on all the variables involved. On the other hand, the behavior of these complexes is governed by a system of Cahn-Hilliard equations featuring reaction terms.
Another intriguing biological phenomenon that has captured my recent interest is chemotaxis, in conjunction with the well-known Keller–Segel model.
Optimal control of PDEs
Optimal control theory aims at finding the smarter choice to
address the solution of a problem (system of PDEs) by minimising suitable quantities which may represent,
in a general sense, some costs. One common choice for such costs is a cost functional of the tracking type, which serves to guide the system toward approximating desired targets.
Typical questions arising in optimal control theory concerns the existence of optimal strategies and optimality conditions for minimisers.
Topological optimisation and Linear elasticity
The combination of the phase-field approach and optimal control theory has proven to be highly effective in addressing problems related to structural topological optimisation.
In this direction, we point out that the basic equations behind those problems are coming from
linear elasticity theory.
A meaningful application concern additive manufacturing.
This is building technique that produces objects
in a layer-by-layer fashion through fusing or binding raw materials in powder and resin
forms.Since its inception in the 1970s, additive manufacturing has demonstrated remarkable versatility, enabling the creation of intricate geometries, rapid modifications, prototyping, and redesigns.
Nonetheless, despite its widespread use in real-world applications, numerous theoretical challenges remain to be resolved.
Dynamic boundary conditions
Dynamic boundary conditions in parabolic partial differential equations are a valuable mathematical tool used to model physical systems where the boundary interactions evolve over time. Unlike traditional boundary conditions like Neumann or Dirichlet, which are typically fixed, dynamic boundary conditions allow for the incorporation of time-dependent effects at the boundary addressing possible intricate dynamics occurring at the boundary.
They find applications in various fields, including heat conduction, fluid dynamics, and diffusion processes, where capturing time-varying boundary effects is essential for accurate modeling and prediction.
Education
Non-tenure track Assistant Professor RTDa (Politecnico di Milano)
July 2022 - Today
Postdoctoral Researcher (University of Pavia)
March 2021 - July 2022
Doctorate in Mathematics (University of Milano-Bicocca)
Title of the Thesis: Understanding the Evolution of Tumours, a Phase-field Approach: Analytic Results and Optimal Control
(supervisor: Prof. Pierluigi Colli).
During my third year of PhD (15/09/19-15/12/19) I had the privilege to be a guest for three months of
Prof. Dr. Harald Garcke at the University of Regensburg.
October 2017 - December 2020
Master of Science in Mathematics (University of Pavia)
Title of the thesis: Boundary control problem and optimality conditions for the Cahn-Hilliard equation with dynamic boundary conditions (supervisor: Prof. Pierluigi Colli), 110/110 cum Laude.
September 2015 - September 2017
Bachelor of Science in Mathematics (University of Pavia)
Title of the thesis: The Legendre-Fenchel transform (supervisor: Prof. Enrico Vitali).
September 2012 - September 2015
Teaching
Politecnico of Milan
Mathematics: degree course in Biomedical Engineering (MEDTEC Program)
A.Y. 2024 - 2025
Mathematics: degree course in Biomedical Engineering (MEDTEC Program)
A.Y. 2023 - 2024
Calculus 2: degree course in civil Engineering
A.Y. 2022 - 2023
Adjunct Professor: Mathematics with elements of Statistic, degree course in Pharmacy (University of Pavia)
A.Y. 2022 - 2023
Exercise lectures: Mathematical and Numerical Methods in Engineering, Master Degree Program in Biomedical Engineering
A.Y. 2022 - 2023
University of Pavia
Adjunct Professor: Mathematics with elements of Statistic, degree course in Pharmacy
A.Y. 2021 - 2022
Exercise lectures: Calculus 2, 4 hours, degree course in Engineering
A.Y. 2020 - 2021
Exercise lectures: Elements of Mathematics and statistic, 12 hours, degree course in Science, technology and environment
A.Y. 2020 - 2021
Seminar lectures: Precorsi, 20 hours, degree course in Engineering
A.Y. 2019 - 2020
Exercise lectures: Advanced Calculus and Statistic, 7 hours, degree course in Engineering
A.Y. 2018 - 2019
Project: Lauree PLS, Il gioco e il Caso, 30 hours
A.Y. 2018 - 2019
Exercise lectures: Calculus 2, 10 hours, degree course in Physics
A.Y. 2018 - 2019
Exercise lectures: Calculus 1, 10 hours, degree course in Engineering
A.Y. 2018 - 2019
Exercise lectures: Elements of Mathematics and statistic, 14 hours, degree course in Science, technology and environment
A.Y. 2018 - 2019
Exercise lectures: Mathematics and statistic, 6 hours, degree course in Biotechnology
A.Y. 2018 - 2019
Exercise lectures: Mathematics, 20 hours, degree course in Biotechnology
A.Y. 2017 - 2018
Exercise lectures: Mathematics, 15 hours, degree course in Biotechnology
A.Y. 2017 - 2018
Exercise lectures: Calculus 2, 28 hours, degree course in Engineering
A.Y. 2016 - 2017
Exercise lectures: Mathematics, 20 hours, degree course in Biotechnology
A.Y. 2015 - 2016
Invited Talks and seminars
- 10/09/2024 Italian-Japanese Workshop on Variational Perspectives for PDEs, "Analysis and optimal control of Keller–Segel–Cahn–Hilliard models for tumor growth", Pavia, Italy.
- 10/06/2024 From Cells to Tissues:
Models, Analysis and Applications: "Exploring RNA-Protein Dynamics through Phase Segregation", Como, Italy.
- 24/05/2024 GRK 2339 IntComSin Kolloquium: "Initiating Phase Separation: Reaction-Driven Solutions
in Cahn–Hilliard Equations", University of Regensburg, Regensburg, Germany.
- 04/03/2024 Dynamics of interfaces: From applied math to physics and material science: "Exploring RNA-Protein Dynamics through Phase Segregation", University of Augsburg, Augsburg, Germany.
- 05/09/2023 XXII Congresso dell'Unione Matematica Italiana: "Chemotaxis model for tumour growth", University of Pisa, Pisa, Italy.
- 02/06/2023 The 13th AIMS Conference on Dynamical Systems, Differential Equations and Applications: "Phase Segregation Drives RNA-Protein Dynamics", University of Wilmington, Wilmington, NC, USA.
- 15/12/2022 Department seminars: "Chemotaxis model for tumour growth", Politecnico di Milano.
- 12/10/2022 MOCETIBI kick-off workshop: "Liquid Droplets in Cell Biology: RNA-Protein model", Sorbonne Université, Paris.
- 23/06/2022 Lake Como School of Advanced Studies - Mathematical models for bio-medical sciences: "Phase segregation in Cell Biology: RNA-Protein model", Como.
- 24/05/2022 INdAM Workshop PHAME2022 - PHAse field MEthods in applied sciences: "Cell’s organisation: RNA-Protein dynamics", Rome.
- 30/09/2021 DMV-ÖMG Annual Conference 2021: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", Universität Passau (Online).
- 13/04/2021 Seminari di Matematica Applicata: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", University of Pavia.
- 25/11/2020 Se mi narri di Matematica: "Mathematical Perspectives on Tumour Growth: From Well-posedness to Optimal Control", University of Pavia.
- 06/02/2020 Insalate di matematica: "Mathematical modeling of cancer: challenges and perspectives towards a tailored therapy", University of Milano-Bicocca.
- 28/11/2019 IntComSin Seminar: "On a phase field model of Cahn-Hilliard type for tumor model with elasticity", Regensburg University.
- 19/11/2019 IntComSin Seminar: "Optimal control theory for parabolic equations: introduction and applications", Regensburg University.
- 02/10/2019 Annual Meeting GRK IntComSin: Interfaces, Complex Structures, and Singular Limits: "Optimal control problems with applications to tumor growth models", Weltenburg Abbey, Kelheim, Germany.
Collaborators
- Dr. Abramo Agosti (Università di Pavia);
- Prof. Pierluigi Colli (University of Pavia);
- Prof. Sergio Frigeri (Università degli Studi di Milano);
- Prof. Gianni Gilardi (University of Pavia);
- Prof. Dr. Harald Garcke (University of Regensburg);
- Prof. Maurizio Grasselli (Politecnico di Milano);
- Prof. Andrew Kei Fong Lam (Hong Kong Baptist University);
- Dr. Patrik Knopf (University of Regensburg);
- Prof. Dr. Robert Nürnberg (University of Trento);
- Dr. Andrea Poiatti (Politecnico di Milano);
- Prof. Elisabetta Rocca (University of Pavia);
- Prof. Luca Scarpa (Politecnico di Milano);
- Prof. Giulio Schimperna (University of Pavia);
- Prof. Dr. Jürgen Sprekels (Weierstrass Institute for Applied Analysis and Stochastics);
My Erdős number is 4 with paths being:
Paul Erdős - Vilmos Komornik - Dan Tiba - Jürgen Sprekels - Signori Andrea,
or
Paul Erdős - Vilmos Komornik - Masahiro Yamamoto - Maurizio Grasselli - Signori Andrea.
The 14th AIMS Conference,
16-20 December 2024,
NYU Abu Dhabi, Abu Dhabi (UAE)
EMS Topical Activity Group (TAG) on Mixtures - Mixtures: Modeling, Analysis and Computing
,
5-7 February 2025,
Prague (Czech Republic)
March-May 2025: Visiting at the University of Regensburg (Humboldt Fellowship program), Germany
10-18 May 2025: Visiting at the Weierstrass Institute (WIAS), Germany
Research interests
phase-field based Tumor growth models
Cancer remains one of the foremost causes of global mortality in our modern era. Undoubtedly, comprehending the intricate process of solid tumor growth stands as one of the principal challenges of the 21st century. Within this context, mathematics holds the potential to assume a pivotal role. Multiscale mathematical modeling offers a quantitative tool that can greatly aid in diagnostic and prognostic applications. This is why nonlinear partial differential equations (PDEs) provide a tangible bridge between the experimental techniques employed by medical professionals and the more abstract realm of mathematics. Numerical solvers can be deployed as supportive tools in clinical therapies. The essence of the phase-field models that pique my interest revolves around the amalgamation of a Cahn–Hilliard type equation featuring a source term (accounting for cell-to-cell adhesion effects) with a reaction-diffusion equation describing the behavior of surrounding species that serve as nutrients.
Biological models and chemotaxis
Recently, phase separation has emerged as a fundamental concept in the field of Cell Biology, particularly concerning
intracellular organization.
In this direction, protein-RNA complexes models play a significant and significant role. These models consist of a protein, two different RNA species, and two distinct protein-RNA complexes. The interaction between the protein and RNA species in a specific solvent is described by a interconnected set of reaction-diffusion equations, with the reaction terms being dependent on all the variables involved. On the other hand, the behavior of these complexes is governed by a system of Cahn-Hilliard equations featuring reaction terms.
Another intriguing biological phenomenon that has captured my recent interest is chemotaxis, in conjunction with the well-known Keller–Segel model.
Optimal control of PDEs
Optimal control theory aims at finding the smarter choice to
address the solution of a problem (system of PDEs) by minimising suitable quantities which may represent,
in a general sense, some costs. One common choice for such costs is a cost functional of the tracking type, which serves to guide the system toward approximating desired targets.
Typical questions arising in optimal control theory concerns the existence of optimal strategies and optimality conditions for minimisers.
Topological optimisation and Linear elasticity
The combination of the phase-field approach and optimal control theory has proven to be highly effective in addressing problems related to structural topological optimisation.
In this direction, we point out that the basic equations behind those problems are coming from
linear elasticity theory.
A meaningful application concern additive manufacturing.
This is building technique that produces objects
in a layer-by-layer fashion through fusing or binding raw materials in powder and resin
forms.Since its inception in the 1970s, additive manufacturing has demonstrated remarkable versatility, enabling the creation of intricate geometries, rapid modifications, prototyping, and redesigns.
Nonetheless, despite its widespread use in real-world applications, numerous theoretical challenges remain to be resolved.
Dynamic boundary conditions
Dynamic boundary conditions in parabolic partial differential equations are a valuable mathematical tool used to model physical systems where the boundary interactions evolve over time. Unlike traditional boundary conditions like Neumann or Dirichlet, which are typically fixed, dynamic boundary conditions allow for the incorporation of time-dependent effects at the boundary addressing possible intricate dynamics occurring at the boundary.
They find applications in various fields, including heat conduction, fluid dynamics, and diffusion processes, where capturing time-varying boundary effects is essential for accurate modeling and prediction.
Education
Non-tenure track Assistant Professor RTDa (Politecnico di Milano)
July 2022 - Today
Postdoctoral Researcher (University of Pavia)
March 2021 - July 2022
Doctorate in Mathematics (University of Milano-Bicocca)
Title of the Thesis: Understanding the Evolution of Tumours, a Phase-field Approach: Analytic Results and Optimal Control
(supervisor: Prof. Pierluigi Colli).
During my third year of PhD (15/09/19-15/12/19) I had the privilege to be a guest for three months of
Prof. Dr. Harald Garcke at the University of Regensburg.
October 2017 - December 2020
Master of Science in Mathematics (University of Pavia)
Title of the thesis: Boundary control problem and optimality conditions for the Cahn-Hilliard equation with dynamic boundary conditions (supervisor: Prof. Pierluigi Colli), 110/110 cum Laude.
September 2015 - September 2017
Bachelor of Science in Mathematics (University of Pavia)
Title of the thesis: The Legendre-Fenchel transform (supervisor: Prof. Enrico Vitali).
September 2012 - September 2015
Teaching
Politecnico of Milan
Mathematics: degree course in Biomedical Engineering (MEDTEC Program)
A.Y. 2024 - 2025
Mathematics: degree course in Biomedical Engineering (MEDTEC Program)
A.Y. 2023 - 2024
Calculus 2: degree course in civil Engineering
A.Y. 2022 - 2023
Adjunct Professor: Mathematics with elements of Statistic, degree course in Pharmacy (University of Pavia)
A.Y. 2022 - 2023
Exercise lectures: Mathematical and Numerical Methods in Engineering, Master Degree Program in Biomedical Engineering
A.Y. 2022 - 2023
University of Pavia
Adjunct Professor: Mathematics with elements of Statistic, degree course in Pharmacy
A.Y. 2021 - 2022
Exercise lectures: Calculus 2, 4 hours, degree course in Engineering
A.Y. 2020 - 2021
Exercise lectures: Elements of Mathematics and statistic, 12 hours, degree course in Science, technology and environment
A.Y. 2020 - 2021
Seminar lectures: Precorsi, 20 hours, degree course in Engineering
A.Y. 2019 - 2020
Exercise lectures: Advanced Calculus and Statistic, 7 hours, degree course in Engineering
A.Y. 2018 - 2019
Project: Lauree PLS, Il gioco e il Caso, 30 hours
A.Y. 2018 - 2019
Exercise lectures: Calculus 2, 10 hours, degree course in Physics
A.Y. 2018 - 2019
Exercise lectures: Calculus 1, 10 hours, degree course in Engineering
A.Y. 2018 - 2019
Exercise lectures: Elements of Mathematics and statistic, 14 hours, degree course in Science, technology and environment
A.Y. 2018 - 2019
Exercise lectures: Mathematics and statistic, 6 hours, degree course in Biotechnology
A.Y. 2018 - 2019
Exercise lectures: Mathematics, 20 hours, degree course in Biotechnology
A.Y. 2017 - 2018
Exercise lectures: Mathematics, 15 hours, degree course in Biotechnology
A.Y. 2017 - 2018
Exercise lectures: Calculus 2, 28 hours, degree course in Engineering
A.Y. 2016 - 2017
Exercise lectures: Mathematics, 20 hours, degree course in Biotechnology
A.Y. 2015 - 2016
Invited Talks and seminars
- 10/09/2024 Italian-Japanese Workshop on Variational Perspectives for PDEs, "Analysis and optimal control of Keller–Segel–Cahn–Hilliard models for tumor growth", Pavia, Italy.
- 10/06/2024 From Cells to Tissues:
Models, Analysis and Applications: "Exploring RNA-Protein Dynamics through Phase Segregation", Como, Italy.
- 24/05/2024 GRK 2339 IntComSin Kolloquium: "Initiating Phase Separation: Reaction-Driven Solutions
in Cahn–Hilliard Equations", University of Regensburg, Regensburg, Germany.
- 04/03/2024 Dynamics of interfaces: From applied math to physics and material science: "Exploring RNA-Protein Dynamics through Phase Segregation", University of Augsburg, Augsburg, Germany.
- 05/09/2023 XXII Congresso dell'Unione Matematica Italiana: "Chemotaxis model for tumour growth", University of Pisa, Pisa, Italy.
- 02/06/2023 The 13th AIMS Conference on Dynamical Systems, Differential Equations and Applications: "Phase Segregation Drives RNA-Protein Dynamics", University of Wilmington, Wilmington, NC, USA.
- 15/12/2022 Department seminars: "Chemotaxis model for tumour growth", Politecnico di Milano.
- 12/10/2022 MOCETIBI kick-off workshop: "Liquid Droplets in Cell Biology: RNA-Protein model", Sorbonne Université, Paris.
- 23/06/2022 Lake Como School of Advanced Studies - Mathematical models for bio-medical sciences: "Phase segregation in Cell Biology: RNA-Protein model", Como.
- 24/05/2022 INdAM Workshop PHAME2022 - PHAse field MEthods in applied sciences: "Cell’s organisation: RNA-Protein dynamics", Rome.
- 30/09/2021 DMV-ÖMG Annual Conference 2021: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", Universität Passau (Online).
- 13/04/2021 Seminari di Matematica Applicata: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", University of Pavia.
- 25/11/2020 Se mi narri di Matematica: "Mathematical Perspectives on Tumour Growth: From Well-posedness to Optimal Control", University of Pavia.
- 06/02/2020 Insalate di matematica: "Mathematical modeling of cancer: challenges and perspectives towards a tailored therapy", University of Milano-Bicocca.
- 28/11/2019 IntComSin Seminar: "On a phase field model of Cahn-Hilliard type for tumor model with elasticity", Regensburg University.
- 19/11/2019 IntComSin Seminar: "Optimal control theory for parabolic equations: introduction and applications", Regensburg University.
- 02/10/2019 Annual Meeting GRK IntComSin: Interfaces, Complex Structures, and Singular Limits: "Optimal control problems with applications to tumor growth models", Weltenburg Abbey, Kelheim, Germany.
Collaborators
- Dr. Abramo Agosti (Università di Pavia);
- Prof. Pierluigi Colli (University of Pavia);
- Prof. Sergio Frigeri (Università degli Studi di Milano);
- Prof. Gianni Gilardi (University of Pavia);
- Prof. Dr. Harald Garcke (University of Regensburg);
- Prof. Maurizio Grasselli (Politecnico di Milano);
- Prof. Andrew Kei Fong Lam (Hong Kong Baptist University);
- Dr. Patrik Knopf (University of Regensburg);
- Prof. Dr. Robert Nürnberg (University of Trento);
- Dr. Andrea Poiatti (Politecnico di Milano);
- Prof. Elisabetta Rocca (University of Pavia);
- Prof. Luca Scarpa (Politecnico di Milano);
- Prof. Giulio Schimperna (University of Pavia);
- Prof. Dr. Jürgen Sprekels (Weierstrass Institute for Applied Analysis and Stochastics);
My Erdős number is 4 with paths being:
Paul Erdős - Vilmos Komornik - Dan Tiba - Jürgen Sprekels - Signori Andrea,
or
Paul Erdős - Vilmos Komornik - Masahiro Yamamoto - Maurizio Grasselli - Signori Andrea.
March-May 2025: Visiting at the University of Regensburg (Humboldt Fellowship program), Germany
10-18 May 2025: Visiting at the Weierstrass Institute (WIAS), Germany
Research interests
phase-field based Tumor growth models
Cancer remains one of the foremost causes of global mortality in our modern era. Undoubtedly, comprehending the intricate process of solid tumor growth stands as one of the principal challenges of the 21st century. Within this context, mathematics holds the potential to assume a pivotal role. Multiscale mathematical modeling offers a quantitative tool that can greatly aid in diagnostic and prognostic applications. This is why nonlinear partial differential equations (PDEs) provide a tangible bridge between the experimental techniques employed by medical professionals and the more abstract realm of mathematics. Numerical solvers can be deployed as supportive tools in clinical therapies. The essence of the phase-field models that pique my interest revolves around the amalgamation of a Cahn–Hilliard type equation featuring a source term (accounting for cell-to-cell adhesion effects) with a reaction-diffusion equation describing the behavior of surrounding species that serve as nutrients.Biological models and chemotaxis
Recently, phase separation has emerged as a fundamental concept in the field of Cell Biology, particularly concerning intracellular organization. In this direction, protein-RNA complexes models play a significant and significant role. These models consist of a protein, two different RNA species, and two distinct protein-RNA complexes. The interaction between the protein and RNA species in a specific solvent is described by a interconnected set of reaction-diffusion equations, with the reaction terms being dependent on all the variables involved. On the other hand, the behavior of these complexes is governed by a system of Cahn-Hilliard equations featuring reaction terms. Another intriguing biological phenomenon that has captured my recent interest is chemotaxis, in conjunction with the well-known Keller–Segel model.Optimal control of PDEs
Optimal control theory aims at finding the smarter choice to address the solution of a problem (system of PDEs) by minimising suitable quantities which may represent, in a general sense, some costs. One common choice for such costs is a cost functional of the tracking type, which serves to guide the system toward approximating desired targets. Typical questions arising in optimal control theory concerns the existence of optimal strategies and optimality conditions for minimisers.Topological optimisation and Linear elasticity
The combination of the phase-field approach and optimal control theory has proven to be highly effective in addressing problems related to structural topological optimisation. In this direction, we point out that the basic equations behind those problems are coming from linear elasticity theory. A meaningful application concern additive manufacturing. This is building technique that produces objects in a layer-by-layer fashion through fusing or binding raw materials in powder and resin forms.Since its inception in the 1970s, additive manufacturing has demonstrated remarkable versatility, enabling the creation of intricate geometries, rapid modifications, prototyping, and redesigns. Nonetheless, despite its widespread use in real-world applications, numerous theoretical challenges remain to be resolved.Dynamic boundary conditions
Dynamic boundary conditions in parabolic partial differential equations are a valuable mathematical tool used to model physical systems where the boundary interactions evolve over time. Unlike traditional boundary conditions like Neumann or Dirichlet, which are typically fixed, dynamic boundary conditions allow for the incorporation of time-dependent effects at the boundary addressing possible intricate dynamics occurring at the boundary. They find applications in various fields, including heat conduction, fluid dynamics, and diffusion processes, where capturing time-varying boundary effects is essential for accurate modeling and prediction.Education
Non-tenure track Assistant Professor RTDa (Politecnico di Milano)
July 2022 - Today
Postdoctoral Researcher (University of Pavia)
March 2021 - July 2022
Doctorate in Mathematics (University of Milano-Bicocca)
Title of the Thesis: Understanding the Evolution of Tumours, a Phase-field Approach: Analytic Results and Optimal Control
(supervisor: Prof. Pierluigi Colli).
During my third year of PhD (15/09/19-15/12/19) I had the privilege to be a guest for three months of
Prof. Dr. Harald Garcke at the University of Regensburg.
During my third year of PhD (15/09/19-15/12/19) I had the privilege to be a guest for three months of
Prof. Dr. Harald Garcke at the University of Regensburg.
October 2017 - December 2020
Master of Science in Mathematics (University of Pavia)
Title of the thesis: Boundary control problem and optimality conditions for the Cahn-Hilliard equation with dynamic boundary conditions (supervisor: Prof. Pierluigi Colli), 110/110 cum Laude.
September 2015 - September 2017
Bachelor of Science in Mathematics (University of Pavia)
Title of the thesis: The Legendre-Fenchel transform (supervisor: Prof. Enrico Vitali).
September 2012 - September 2015
Teaching
Politecnico of Milan
Mathematics: degree course in Biomedical Engineering (MEDTEC Program)
A.Y. 2024 - 2025
Mathematics: degree course in Biomedical Engineering (MEDTEC Program)
A.Y. 2023 - 2024
Calculus 2: degree course in civil Engineering
A.Y. 2022 - 2023
Adjunct Professor: Mathematics with elements of Statistic, degree course in Pharmacy (University of Pavia)
A.Y. 2022 - 2023
Exercise lectures: Mathematical and Numerical Methods in Engineering, Master Degree Program in Biomedical Engineering
A.Y. 2022 - 2023
University of Pavia
Adjunct Professor: Mathematics with elements of Statistic, degree course in Pharmacy
A.Y. 2021 - 2022
Exercise lectures: Calculus 2, 4 hours, degree course in Engineering
A.Y. 2020 - 2021
Exercise lectures: Elements of Mathematics and statistic, 12 hours, degree course in Science, technology and environment
A.Y. 2020 - 2021
Seminar lectures: Precorsi, 20 hours, degree course in Engineering
A.Y. 2019 - 2020
Exercise lectures: Advanced Calculus and Statistic, 7 hours, degree course in Engineering
A.Y. 2018 - 2019
Project: Lauree PLS, Il gioco e il Caso, 30 hours
A.Y. 2018 - 2019
Exercise lectures: Calculus 2, 10 hours, degree course in Physics
A.Y. 2018 - 2019
Exercise lectures: Calculus 1, 10 hours, degree course in Engineering
A.Y. 2018 - 2019
Exercise lectures: Elements of Mathematics and statistic, 14 hours, degree course in Science, technology and environment
A.Y. 2018 - 2019
Exercise lectures: Mathematics and statistic, 6 hours, degree course in Biotechnology
A.Y. 2018 - 2019
Exercise lectures: Mathematics, 20 hours, degree course in Biotechnology
A.Y. 2017 - 2018
Exercise lectures: Mathematics, 15 hours, degree course in Biotechnology
A.Y. 2017 - 2018
Exercise lectures: Calculus 2, 28 hours, degree course in Engineering
A.Y. 2016 - 2017
Exercise lectures: Mathematics, 20 hours, degree course in Biotechnology
A.Y. 2015 - 2016
Invited Talks and seminars
- 10/09/2024 Italian-Japanese Workshop on Variational Perspectives for PDEs, "Analysis and optimal control of Keller–Segel–Cahn–Hilliard models for tumor growth", Pavia, Italy.
- 10/06/2024 From Cells to Tissues: Models, Analysis and Applications: "Exploring RNA-Protein Dynamics through Phase Segregation", Como, Italy.
- 24/05/2024 GRK 2339 IntComSin Kolloquium: "Initiating Phase Separation: Reaction-Driven Solutions in Cahn–Hilliard Equations", University of Regensburg, Regensburg, Germany.
- 04/03/2024 Dynamics of interfaces: From applied math to physics and material science: "Exploring RNA-Protein Dynamics through Phase Segregation", University of Augsburg, Augsburg, Germany.
- 05/09/2023 XXII Congresso dell'Unione Matematica Italiana: "Chemotaxis model for tumour growth", University of Pisa, Pisa, Italy.
- 02/06/2023 The 13th AIMS Conference on Dynamical Systems, Differential Equations and Applications: "Phase Segregation Drives RNA-Protein Dynamics", University of Wilmington, Wilmington, NC, USA.
- 15/12/2022 Department seminars: "Chemotaxis model for tumour growth", Politecnico di Milano.
- 12/10/2022 MOCETIBI kick-off workshop: "Liquid Droplets in Cell Biology: RNA-Protein model", Sorbonne Université, Paris.
- 23/06/2022 Lake Como School of Advanced Studies - Mathematical models for bio-medical sciences: "Phase segregation in Cell Biology: RNA-Protein model", Como.
- 24/05/2022 INdAM Workshop PHAME2022 - PHAse field MEthods in applied sciences: "Cell’s organisation: RNA-Protein dynamics", Rome.
- 30/09/2021 DMV-ÖMG Annual Conference 2021: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", Universität Passau (Online).
- 13/04/2021 Seminari di Matematica Applicata: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", University of Pavia.
- 25/11/2020 Se mi narri di Matematica: "Mathematical Perspectives on Tumour Growth: From Well-posedness to Optimal Control", University of Pavia.
- 06/02/2020 Insalate di matematica: "Mathematical modeling of cancer: challenges and perspectives towards a tailored therapy", University of Milano-Bicocca.
- 28/11/2019 IntComSin Seminar: "On a phase field model of Cahn-Hilliard type for tumor model with elasticity", Regensburg University.
- 19/11/2019 IntComSin Seminar: "Optimal control theory for parabolic equations: introduction and applications", Regensburg University.
- 02/10/2019 Annual Meeting GRK IntComSin: Interfaces, Complex Structures, and Singular Limits: "Optimal control problems with applications to tumor growth models", Weltenburg Abbey, Kelheim, Germany.
Collaborators
- Dr. Abramo Agosti (Università di Pavia);
- Prof. Pierluigi Colli (University of Pavia);
- Prof. Sergio Frigeri (Università degli Studi di Milano);
- Prof. Gianni Gilardi (University of Pavia);
- Prof. Dr. Harald Garcke (University of Regensburg);
- Prof. Maurizio Grasselli (Politecnico di Milano);
- Prof. Andrew Kei Fong Lam (Hong Kong Baptist University);
- Dr. Patrik Knopf (University of Regensburg);
- Prof. Dr. Robert Nürnberg (University of Trento);
- Dr. Andrea Poiatti (Politecnico di Milano);
- Prof. Elisabetta Rocca (University of Pavia);
- Prof. Luca Scarpa (Politecnico di Milano);
- Prof. Giulio Schimperna (University of Pavia);
- Prof. Dr. Jürgen Sprekels (Weierstrass Institute for Applied Analysis and Stochastics);
My Erdős number is 4 with paths being: Paul Erdős - Vilmos Komornik - Dan Tiba - Jürgen Sprekels - Signori Andrea,
or
Paul Erdős - Vilmos Komornik - Masahiro Yamamoto - Maurizio Grasselli - Signori Andrea.