Andrea Signori, PhD, AvH Fellow

Dipartimento di Matematica • Politecnico di Milano • Via E. Bonardi 9, 20133 Milano, Italy • andrea.signori@polimi.it

Pubblicazioni (Ultimo aggiornamento: 14/03/25, ordine cronologico inverso)

Preprints Recenti

  1. M. Grasselli, L. Melzi and A. Signori, On a non-local phase-field model for tumour growth with single-well Lennard–Jones potential.
    Preprint arXiv:2503.10495 [math.AP], (2025), 1-28.
  2. H. Garcke, K. F. Lam, R. Nürnberg and A. Signori, On a Cahn–Hilliard equation for the growth and division of chemically active droplets modeling protocells.
    Preprint arXiv:2503.09581 [math.AP], (2025), 1-33.
  3. H. Garcke, P. Knopf and A.Signori, The anisotropic Cahn–Hilliard equation with degenerate mobility: Existence of weak solutions.
    Preprint arXiv:2502.13799 [math.AP], (2025), 1-20.
  4. P. Colli, G. Gilardi, A. Signori and J. Sprekels, Solvability and optimal control of a multi-species Cahn–Hilliard–Keller–Segel tumor growth model.
    Preprint arXiv:2407.18162 [math.AP], (2024), 1-39.    WIAS Preprint.
  5. M. Grasselli, L. Scarpa and A. Signori, Cahn–Hilliard equations with singular potential, reaction term and pure phase initial datum.
    Preprint arXiv:2404.12113 [math.AP], (2024), 1-32.

Papers Pubblicati

  1. P. Colli, P. Knopf, G. Schimperna and A. Signori, Two-phase flows through porous media described by a Cahn–Hilliard–Brinkman model with dynamic boundary conditions.
    J. Evol. Equ., 24(85) (2024), Online first.
    doi.org/10.1007/s00028-024-00999-y.    Preprint arXiv:2312.15274 [math.AP]
  2. H. Garcke, K. F. Lam, R. Nürnberg and A. Signori, Complex pattern formation governed by a Cahn–Hilliard–Swift–Hohenberg system: Analysis and numerical simulations.
    Math. Models Methods Appl. Sci., 34(11) (2024), 2055-2097.
    doi.org/10.1142/S021820252450043X.    Preprint arXiv:2405.01947 [math.AP]
  3. P. Colli, G. Gilardi, A. Signori and J. Sprekels, Curvature effects in pattern formation: well-posedness and optimal control of a sixth-order Cahn–Hilliard equation.
    SIAM J. Math. Anal., 56 (2024), 4253-5078.
    doi.org/10.1137/24M1630372.    Preprint arXiv:2401.05189 [math.AP].    WIAS Preprint.
  4. A. Agosti and A. Signori, Analysis of a multi-species Cahn–Hilliard–Keller–Segel tumor growth model with chemotaxis and angiogenesis.
    J. Differential Equations, 403 (2024), 308-367.
    doi.org/10.1016/j.jde.2024.05.025.    Preprint arXiv:2311.13470 [math.AP]
  5. A. Poiatti and A. Signori, Regularity results and optimal velocity control of the convective nonlocal Cahn–Hilliard equation in 3D.
    ESAIM Control Optim. Calc. Var., 30 (Online first) (2024).
    doi.org/10.1051/cocv/2024007.    Preprint arXiv:2304.12074 [math.OC]
  6. P. Colli, G. Gilardi, A. Signori and J. Sprekels, On a Cahn–Hilliard system with source term and thermal memory.
    Nonlinear Analysis, 240 (2023), 113461.
    doi.org/10.1016/j.na.2023.113461.    Preprint arXiv:2207.08491 [math.AP].    WIAS Preprint.
  7. P. Colli, G. Gilardi, A. Signori and J. Sprekels, Optimal temperature distribution for a nonisothermal Cahn–Hilliard system in two dimensions with source term and double obstacle potential.
    Ann. Acad. Rom. Sci., Ser. Math. Appl., 15 (2023), 175-204.
    doi.org/10.56082/annalsarscimath.2023.1-2.175.    Preprint arXiv:2303.13266 [math.OC].    WIAS Preprint.
  8. G. Gilardi, E. Rocca and A. Signori,
    Well-posedness and optimal control for a viscous Cahn–Hilliard–Oono system with dynamic boundary conditions.
    Discrete Contin. Dyn. Syst. Ser. S, 16 (2023), 3573-3605.
    doi/10.3934/dcdss.2023127.    Preprint arXiv:2309.09053 [math.AP].
  9. G. Gilardi, A. Signori and J. Sprekels,
    Nutrient control for a viscous Cahn–Hilliard–Keller–Segel model with logistic source describing tumor growth.
    Discrete Contin. Dyn. Syst. Ser. S, 16 (2023), 3552-3572.
    doi/10.3934/dcdss.2023123.    Preprint arXiv:2309.09052 [math.OC]
  10. P. Colli, G. Gilardi, A. Signori and J. Sprekels, Optimal temperature distribution for a nonisothermal Cahn–Hilliard system with source term.
    Appl. Math. Optim., 88, Online first (2023).
    doi.org/10.1007/s00245-023-10039-9.    Preprint arXiv:2303.00488 [math.OC].    WIAS Preprint.
  11. P. Colli, G. Gilardi, A. Signori and J. Sprekels, Cahn–Hilliard–Brinkman model for tumor growth with possibly singular potentials.
    Nonlinearity, 36 (2023), 4470-4500.
    doi.org/10.1088/1361-6544/ace2a7.    Preprint arXiv:2204.13526 [math.AP].    WIAS Preprint.
  12. H. Garcke, K. F. Lam, R. Nürnberg and A. Signori, Phase field topology optimisation for 4D printing.
    ESAIM Control Optim. Calc. Var., Online first (2023).
    doi.org/10.1051/cocv/2023012.    Preprint arXiv:2207.03706 [math.OC].
  13. H. Garcke, K. F. Lam, R. Nürnberg and A. Signori, Overhang penalization in additive manufacturing via phase field structural topology optimization with anisotropic energies.
    Appl. Math. Optim., 87(44) (2023).
    doi.org/10.1007/s00245-022-09939-z.    Preprint arXiv:2111.14070 [math.OC].
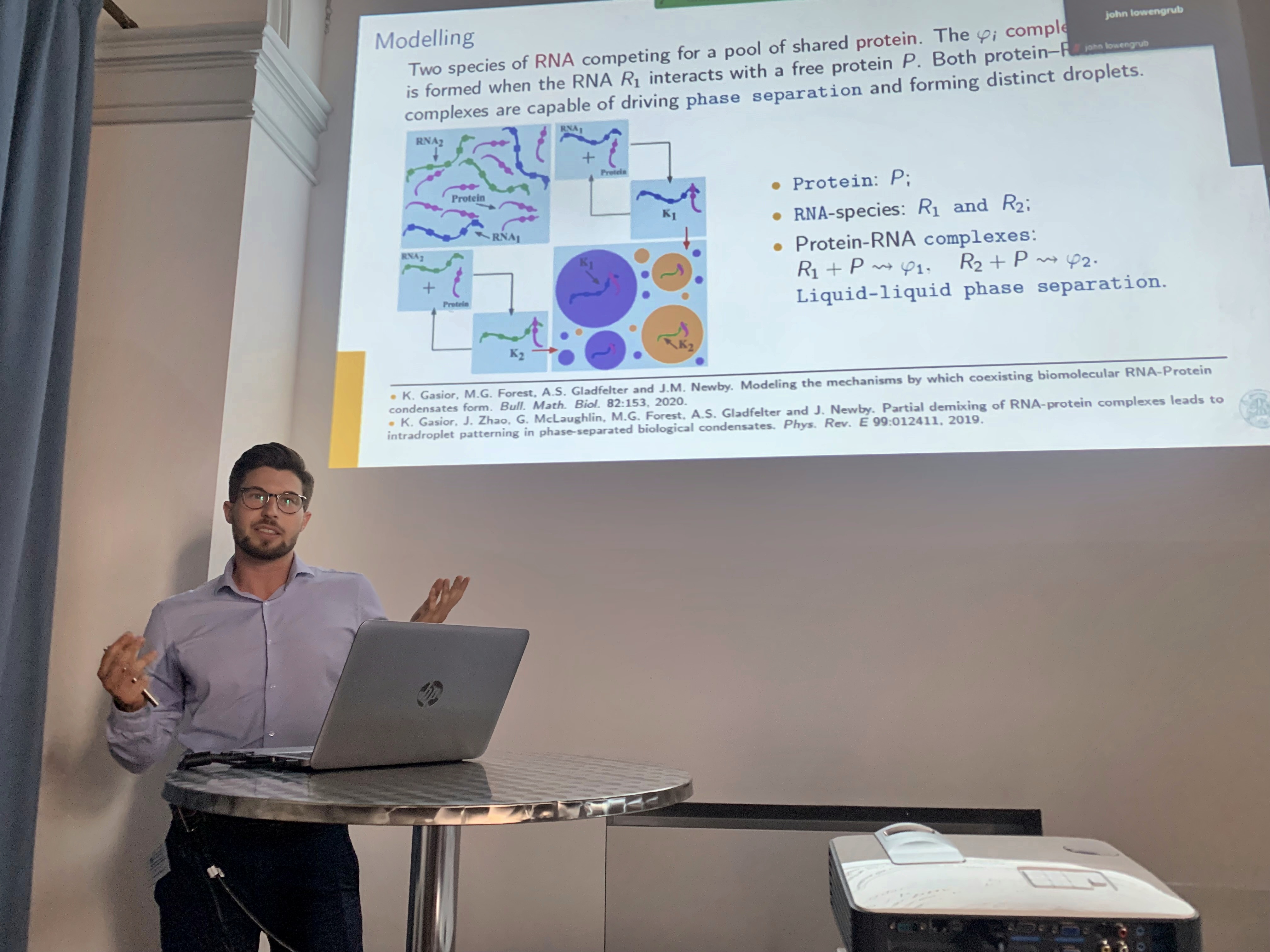
  14. M. Grasselli, L. Scarpa and A. Signori, On a phase field model for RNA-Protein dynamics.
    SIAM J. Math. Anal., 55(1) (2023), 405-457.
    doi.org/10.1137/22M1483086.    Preprint arXiv:2203.03258 [math.AP].
  15. P. Colli, G. Gilardi, A. Signori and J. Sprekels, Optimal control of a nonconserved phase field model of Caginalp type with thermal memory and double obstacle potential.
    Discrete Contin. Dyn. Syst. Ser. S, 16(9) (2023), 2305-2325.
    doi.org/10.3934/dcdss.2022210.    Preprint arXiv:2207.00375 [math.OC].    WIAS Preprint.
  16. E. Rocca, G. Schimperna and A. Signori, On a Cahn–Hilliard–Keller–Segel model with generalized logistic source describing tumor growth.
    J. Differential Equations, 343 (2023), 530-578.
    doi.org/10.1016/j.jde.2022.10.026.    Preprint arXiv:2202.11007 [math.AP].
  17. P. Colli, A. Signori and J. Sprekels, Analysis and optimal control theory for a phase field model of Caginalp type with thermal memory.
    Commun. Optim. Theory, 4 (2022).
    doi.org/10.23952/cot.2022.4.    Preprint arXiv:2107.09565 [math.OC].    WIAS Preprint.
  18. P. Colli, A. Signori and J. Sprekels, Optimal control problems with sparsity for phase field tumor growth models involving variational inequalities. J. Optim. Theory Appl., 194 (2022), 25-58.
    doi.org/10.1007/s10957-022-02000-7.    Preprint arXiv:2104.09814 [math.OC].    WIAS Preprint.
  19. E. Rocca, L. Scarpa and A. Signori, Parameter identification for nonlocal phase field models for tumor growth via optimal control and asymptotic analysis. Math. Models Methods Appl. Sci., 31(13) (2021), 2643-2694.
    doi.org/10.1142/S0218202521500585.    Preprint arXiv:2009.11159 [math.AP].
  20. P. Knopf and A. Signori, Existence of weak solutions to multiphase Cahn–Hilliard–Darcy and Cahn–Hilliard–Brinkman models for stratified tumor growth with chemotaxis and general source terms. Comm. Partial Differential Equations, 47(2) (2022), 233-278.
    doi.org/10.1080/03605302.2021.1966803.    Preprint arXiv:2105.09068 [math.AP].
  21. P. Colli, A. Signori and J. Sprekels, Second-order analysis of an optimal control problem in a phase field tumor growth model with singular potentials and chemotaxis. ESAIM Control Optim. Calc. Var., 27 (2021).
    doi.org/10.1051/cocv/2021072.    Preprint arXiv:2009.07574 [math.AP].    WIAS Preprint.
  22. L. Scarpa and A. Signori, On a class of non-local phase-field models for tumor growth with possibly singular potentials, chemotaxis, and active transport. Nonlinearity, 34 (2021), 3199-3250.
    doi.org/10.1088/1361-6544/abe75d.    Preprint arXiv:2002.12702 [math.AP].
  23. H. Garcke, K. F. Lam and A. Signori, Sparse optimal control of a phase field tumour model with mechanical effects.
    SIAM J. Control Optim., 59(2) (2021), 1555-1580.
    doi.org/10.1137/20M1372093.    Preprint arXiv:2010.03767 [math.OC].
  24. S. Frigeri, K. F. Lam and A. Signori, Strong well-posedness and inverse identification problem of a non-local phase field tumor model with degenerate mobilities. European J. Appl. Math., 33(2) (2022), 267-308.
    doi:10.1017/S0956792521000012.    Preprint arXiv:2004.04537 [math.AP].
  25. P. Knopf and A. Signori, On the nonlocal Cahn–Hilliard equation with nonlocal dynamic boundary condition and boundary penalization.
    J. Differential Equations, 280(4) (2021), 236-291.
    doi.org/10.1016/j.jde.2021.01.012.    Preprint arXiv:2004.00093 [math.AP].
  26. H. Garcke, K. F. Lam and A. Signori, On a phase field model of Cahn–Hilliard type for tumour growth with mechanical effects.
    Nonlinear Anal. Real World Appl., 57 (2021), 103192.
    doi.org/10.1016/j.nonrwa.2020.103192.    Preprint arXiv:1912.01945 [math.AP].
  27. P. Colli, A. Signori and J. Sprekels, Optimal control of a phase field system modelling tumor growth with chemotaxis and singular potentials.
    Appl. Math. Optim., 83 (2021), 2017-2049.
    doi.org/10.1007/s00245-019-09618-6   (see also the Erratum).    Preprint arXiv:1907.03566 [math.AP].    WIAS Preprint.
  28. P. Colli and A. Signori, Boundary control problem and optimality conditions for the Cahn–Hilliard equation with dynamic boundary conditions.
    Internat. J. Control, 94 (2021), 1852-1869.
    doi.org/10.1080/00207179.2019.1680870.    Preprint arXiv:1905.00203 [math.AP].
  29. A. Signori, Penalisation of long treatment time and optimal control of a tumour growth model of Cahn–Hilliard type with singular potential.
    Discrete Contin. Dyn. Syst. Ser. A, 41(6) (2021), 2519-2542.
    doi.org/10.3934/dcds.2020373.    Preprint arXiv:1906.03460 [math.AP].
  30. A. Signori, Vanishing parameter for an optimal control problem modeling tumor growth.
    Asymptot. Anal., 117 (2020), 43-66.
    doi.org/10.3233/ASY-191546.    Preprint arXiv:1903.04930 [math.AP].
  31. A. Signori, Optimal treatment for a phase field system of Cahn–Hilliard type modeling tumor growth by asymptotic scheme.
    Math. Control Relat. Fields, 10 (2020), 305-331.
    doi:10.3934/mcrf.2019040.    Preprint arXiv:1902.01079 [math.AP].
  32. A. Signori, Optimality conditions for an extended tumor growth model with double obstacle potential via deep quench approach.
    Evol. Equ. Control Theory, 9(1) (2020), 193-217.
    doi:10.3934/eect.2020003.    Preprint arXiv:1811.08626 [math.AP].
  33. A. Signori, Optimal distributed control of an extended model of tumor growth with logarithmic potential.
    Appl. Math. Optim., 82 (2020), 517-549.
    doi.org/10.1007/s00245-018-9538-1.    Preprint arXiv:1809.06834 [math.AP].

  34. PhD Thesis: A. Signori, Understanding the Evolution of Tumours, a Phase-field Approach: Analytic Results and Optimal Control, 2020.
    (Doctoral advisor: Prof. Pierluigi Colli, Università di Pavia).

Educazione

  

Ricercatore a tempo determinato di tipo a (Politecnico di Milano)

Luglio 2022 - Oggi

Assegnista di Ricerca (Università di Pavia)

Marzo 2021 - Luglio 2022

Dottorato in Matematica (Università di Milano-Bicocca)

Joint PhD Program in Mathematics Pavia-Milano-Bicocca-INdaM
Titolo della Tesi: Understanding the Evolution of Tumours, a Phase-field Approach: Analytic Results and Optimal Control (relatore: Prof. Pierluigi Colli).

Durante il mio terzo anno di dottorato (15/09/19-15/12/19) ho avuto il privilegio di essere ospite per tre mesi del
Prof. Dr. Harald Garcke presso l'Università di Ratisbona.
Ottobre 2017 - Dicembre 2020

Laurea Magistrale in Matematica (Università di Pavia)

Titolo della Tesi: Boundary control problem and optimality conditions for the Cahn-Hilliard equation with dynamic boundary conditions (relatore: Prof. Pierluigi Colli), 110/110 cum Laude.

Settembre 2015 - Settembre 2017

Laurea Triennale in Matematica (Università di Pavia)

Titolo della Tesi: The Legendre-Fenchel transform (relatore: Prof. Enrico Vitali).

Settembre 2012 - Settembre 2015

Didattica


Politecnico di Milano


Mathematics: corso di laurea in Ingegneria Biomedica (MEDTEC Program)

A.Y. 2024 - 2025

Mathematics: corso di laurea in Ingegneria Biomedica (MEDTEC Program)

A.Y. 2023 - 2024

Analisi 2: corso di laurea in Ingegneria Civile

A.A. 2022 - 2023

Professore a Contratto: Matematica con Elementi di Statistica, corso di laurea in Farmacia (Università degli studi di Pavia)

A.A. 2022 - 2023

Esercitazioni (EN): Mathematical and Numerical Methods in Engineering, Master Degree Program in Biomedical Engineering

A.A. 2022 - 2023

Università degli studi di Pavia


Professore a Contratto: Matematica con Elementi di Statistica, corso di laurea in Farmacia

A.A. 2021 - 2022

Seminari didattici: Complementi di Analisi 2, 4 ore, corso di laurea in Ingegneria

A.A. 2020 - 2021

Seminari didattici: Elementi di Matematica e Statistica, 12 ore, corso di laurea in Scienze e Tecnologie per l'Ambiente e la Natura

A.A. 2020- 2021

Seminari didattici: Videolezioni precorsi, 20 ore, corso di laurea in Ingegneria

A.A. 2019 - 2020

Seminari didattici: Complementi di Analisi Matematica e Statistica, 7 ore, corso di laurea in Ingegneria

A.A. 2018 - 2019

Progetto Lauree PLS, Il gioco e il Caso, 30 ore

A.A. 2018 - 2019

Seminari didattici: Complementi di Analisi 2, 10 ore, corso di laurea in Fisica

A.A. 2018 - 2019

Seminari didattici: Analisi 1, 10 ore, corso di laurea in Ingegneria

A.A. 2018 - 2019

Seminari didattici: Elementi di Matematica e Statistica, 14 ore, corso di laurea in Scienze e Tecnologie per l'Ambiente e la Natura

A.A. 2018 - 2019

Seminari didattici: Matematica e Statistica, 6 ore, corso di laurea in Biotecnologie

A.A. 2018 - 2019

Tutorato: Sostegno all’apprendimento per insegnamenti scelti in ambito matematico, 20 ore, corso di laurea in Biotecnologie

A.A. 2017 - 2018

Tutorato: Sostegno al superamento esame, 15 ore, corso di laurea in Biologie

A.A. 2017 - 2018

Tutorato: Analisi matematica 2, 28 ore, corso di laurea in Ingegneria

A.A. 2016 - 2017

Tutorato: Sostegno all’apprendimento per insegnamenti scelti in ambito matematico, 20 ore, corso di laurea in Biotecnologie

A.A. 2015 - 2016

Talks e seminari su invito



  • 25/04/2025   GRK 2339 IntComSin Kolloquium: "Non-Equilibrium Droplets: Mathematical Approaches to Active Phase Separation", University of Regensburg, Germany.
  • 02/04/2025   PDE Afternoon University of Wien, "Active Phase Separation: A Mathematical Perspective on Droplet Dynamics", University of Wien, Vienna, Austria.
  • 06/02/2025   EMS Topical Activity Group (TAG) on Mixtures: - Mixtures: Modeling, Analysis and Computing, "Dynamics of active droplet formation in reaction-driven Cahn–Hilliard models", Charles University, Prague, Czech Republic.
  • 18/12/2024   The 14th AIMS Conference, SS24: Optimal control and parameter estimation in biological models, "Optimal control of Cahn–Hilliard–Keller–Segel tumor growth models", NYU Abu Dhabi, United Arab Emirates.
  • 18/12/2024   The 14th AIMS Conference, SS10: Analysis of diffuse and sharp interface models, "Active droplet formation in Cahn–Hilliard models with chemical reactions", NYU Abu Dhabi, United Arab Emirates.
  • 10/09/2024   Italian-Japanese Workshop on Variational Perspectives for PDEs, "Analysis and optimal control of Keller–Segel–Cahn–Hilliard models for tumor growth", Pavia, Italy.
  • 11/07/2024   C.I.M.E. Diffuse Interface methods in Continuum Mechanics: analysis, singular limits and algorithms, "Triggering phase dynamics starting from a pure phase", Cetraro, Italy.
  • 10/06/2024   From Cells to Tissues: Models, Analysis and Applications: "Exploring RNA-Protein Dynamics through Phase Segregation", Como, Italy.
  • 24/05/2024   GRK 2339 IntComSin Kolloquium: "Initiating Phase Separation: Reaction-Driven Solutions in Cahn–Hilliard Equations", University of Regensburg, Regensburg, Germany.
  • 04/03/2024   Dynamics of interfaces: From applied math to physics and material science: "Exploring RNA-Protein Dynamics through Phase Segregation", University of Augsburg, Augsburg, Germany.
  • 05/09/2023   XXII Congresso dell'Unione Matematica Italiana: "Chemotaxis model for tumour growth", Università di Pisa, Pisa, Italia.
  • 02/06/2023   The 13th AIMS Conference on Dynamical Systems, Differential Equations and Applications: "Phase Segregation Drives RNA-Protein Dynamics", University of Wilmington, Wilmington, NC, USA.
  • 15/12/2022   Seminari dipartimentali: "Chemotaxis model for tumour growth", Politecnico di Milano.
  • 12/10/2022   MOCETIBI kick-off workshop: "Liquid Droplets in Cell Biology: RNA-Protein model", Sorbonne Université, Parigi.
  • 23/06/2022   Lake Como School of Advanced Studies - Mathematical models for bio-medical sciences: "Phase segregation in Cell Biology: RNA-Protein model", Como.
  • 24/05/2022   INdAM Workshop PHAME2022 - PHAse field MEthods in applied sciences: "Cell’s organisation: RNA-Protein dynamics", Roma.
  • 30/09/2021   DMV-ÖMG Annual Conference 2021: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", Universität Passau (Online).
  • 04/13/2021   Seminari di Matematica Applicata: "Mechanical model for tumour growth: mathematical analysis and optimal therapies", Università di Pavia.
  • 25/11/2020   Se mi narri di Matematica: "Mathematical Perspectives on Tumour Growth: From Well-posedness to Optimal Control", Università di Pavia.
  • 06/02/2020   Insalate di matematica: "Mathematical modeling of cancer: challenges and perspectives towards a tailored therapy", Università di Milano-Bicocca.
  • 28/11/2019   IntComSin Seminar: "On a phase field model of Cahn-Hilliard type for tumor model with elasticity", Università di Ratisbona.
  • 19/11/2019   IntComSin Seminar: "Optimal control theory for parabolic equations: introduction and applications", Università di Ratisbona.
  • 02/10/2019   Annual Meeting GRK IntComSin: Interfaces, Complex Structures, and Singular Limits: "Optimal control problems with applications to tumor growth models", Weltenburg Abbey, Kelheim, Germania.

Collaboratori



    Research line: Evolution PDEs, Optimal control and game theory



Il mio numero di Erdős è 4 dato da: Paul Erdős - Vilmos Komornik - Dan Tiba - Jürgen Sprekels - Signori Andrea,
oppure
Paul Erdős - Vilmos Komornik - Masahiro Yamamoto - Maurizio Grasselli - Signori Andrea.



Privacy Policy    Cookie Policy